




Next: Properties of Fourier transforms
Up: Fourier analysis of periodic
Previous: Periodicity of the Fourier
Contents
Index
Fourier transform as additive synthesis
Now consider an arbitrary signal ![$X[n]$](img664.png) that repeats every
that repeats every  samples. (Previously we
had assumed that
samples. (Previously we
had assumed that ![$X[n]$](img664.png) could be obtained as a sum of sinusoids, but we haven't
yet found out whether every periodic
could be obtained as a sum of sinusoids, but we haven't
yet found out whether every periodic ![$X[n]$](img664.png) can be obtained that way.) Let
can be obtained that way.) Let
![$Y[k]$](img1063.png) denote the Fourier transform of
denote the Fourier transform of  for
for
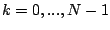 :
:
In the second version we rearranged the exponents to show that ![$Y[k]$](img1063.png) is
a sum of complex sinusoids, with complex amplitudes
is
a sum of complex sinusoids, with complex amplitudes
![$X[n]$](img664.png) and frequencies
and frequencies 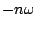 for
for
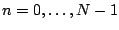 . In other words,
. In other words,
![$Y[k]$](img1063.png) can be considered as a waveform in its own right, whose
can be considered as a waveform in its own right, whose 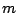 th component
has strength
th component
has strength ![$X[-m]$](img1069.png) . We can also express the amplitude of the partials of
. We can also express the amplitude of the partials of
![$Y[k]$](img1063.png) using its own Fourier transform. Equating the two gives:
using its own Fourier transform. Equating the two gives:
(The expression ![$X[-m]$](img1069.png) makes sense because
makes sense because  is a periodic signal). This
means in turn that
is a periodic signal). This
means in turn that ![$X[-m]$](img1069.png) can be obtained by summing sinusoids with
amplitudes
can be obtained by summing sinusoids with
amplitudes ![$Y[k]/N$](img1071.png) . Replacing
. Replacing 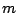 with its negative gives:
with its negative gives:
This shows that any periodic ![$X[n]$](img664.png) can indeed be obtained as a sum of
sinusoids. Further, it explicitly shows how to reconstruct
can indeed be obtained as a sum of
sinusoids. Further, it explicitly shows how to reconstruct ![$X[n]$](img664.png) from its
Fourier transform
from its
Fourier transform ![$Y[k]$](img1063.png) , if we know its value for the integers
, if we know its value for the integers
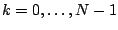 .
.





Next: Properties of Fourier transforms
Up: Fourier analysis of periodic
Previous: Periodicity of the Fourier
Contents
Index
Miller Puckette
2006-09-05
![]() that repeats every
that repeats every ![]() samples. (Previously we
had assumed that
samples. (Previously we
had assumed that ![]() could be obtained as a sum of sinusoids, but we haven't
yet found out whether every periodic
could be obtained as a sum of sinusoids, but we haven't
yet found out whether every periodic ![]() can be obtained that way.) Let
can be obtained that way.) Let
![]() denote the Fourier transform of
denote the Fourier transform of ![]() for
for
![]() :
: