One-pole low-pass filter

Figure 8.12: One-pole lowpass filter: (a) pole-zero diagram; (b) frequency response.

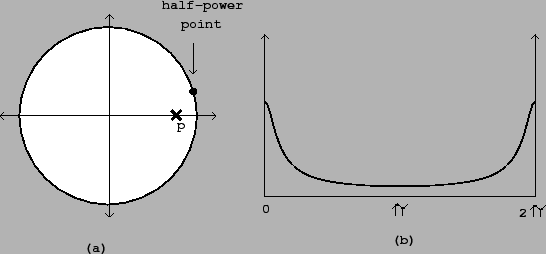
The one-pole low-pass filter has a single pole located at a positive real number $p$, as pictured in Figure 8.12. This is just a recirculating comb filter with delay length $d=1$, and the analysis of section 7.4 applies. The maximum gain occurs at a frequency of zero, corresponding to the point on the circle closest to the point $p$. The gain there is $1/(1-p)$. If we move a distance of $1-p$ units up or down from the real (horizontal) axis, the distance increases by a factor of about $\sqrt{2}$, and so we expect the half-power point to occur at an angular frequency of about $1-p$.

This calculation is often made in reverse: if we wish the half-power point to lie at a given angular frequency $\omega$, we set $p = 1-\omega$. This approximation only works well if the value of $\omega$ is well under $\pi /2$, as it usually is in practice.

It is customary to normalize the one-pole lowpass filter, multiplying it by the constant factor $1-p$ in order to give a gain of 1 at zero frequency; nonzero freqencies will then get a gain less than one.

The frequency response is graphed in Figure 8.12 part (b). The audible frequencies only reach to the middle of the graph; the right-hand side of the frequency response curve all lies above the Nyquist frequency $\pi$.

The one-pole lowpass filter is often used to smooth noisy signals to seek slow-moving trends in them. For instance, if you use a physical controller and care about changes on the order of 1/10 second or so, you can smooth the control by using a low-pass filter whose half-power point is 20 or 30 cycles per second.